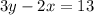Answer:
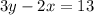
Explanation:
Given
Points (-14,-5) and (4,7)
Required
Find its linear equation in a standard form
To find the linear form, we start by calculating the slope of the line
This is calculated as thus:

Where
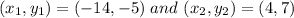
So,
 becomes
becomes
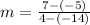
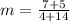

Simplify fraction to lowest term
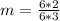
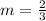
The equation of the line can then be calculated using any of the given points;
Using
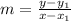
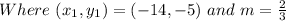
We have
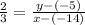
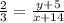
Multiply both sides by 3
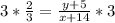


Multiply both sides by x + 14

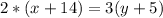
Open brackets

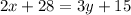
Subtract 2x from both sides
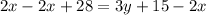
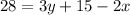
Subtract 15 from both sides

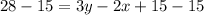
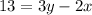
Reorder
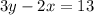
Hence, the equation of the line in standard form is