Question

A standing wave on a string that is fixed at both ends has frequency 80.0 Hz. The distance between adjacent antinodes of the standing wave is 12.0 cm. What is the speed of the waves on the string, in m/s

Answer 1

v = 19.2 m/s

Step-by-step explanation:

In order to find the speed of the string you use the following formula:

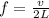`f=(v)/(2L)` (1)

f: frequency of the string = 80.0Hz

v: speed of the wave = ?

L: length of the string = 12.0cm = 0.12m

The length of the string coincides with the wavelength of the wave for the fundamental mode.

Then, you solve for v in the equation (1), and replace the values of the other parameters:

`v=2Lf=2(0.12m)(80.0Hz)=19.2(m)/(s)`

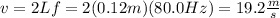
The speed of the wave is 19.2 m/s