Question

Solve using any appropriate method. If the system has an infinite number of solutions, use set-builder notation to write

the solution set. If the system has no solution, state this
13) 4x + 8y = 8
5x + 7y = 1
A) (-8,6)
B) (4,3)
C) (-4,3)
D) No solution
14) 7x + 7y = 8
5x + 5y = 10
A) (10,5)
D) (1,1)
C) {(x,y)|7x + 7y = 8)
B) No solution
15) y = 3x + 4
5x + y = -92
A) No solution
C) (4,8)
B) ((x, y) 5x + y = -92)
D) (-4,-8)
16) 0.4x + 0.4y = 4
0.4x - 0.4y = 1
D)
B)
(659
0(*)
C) (1.4)
D) {x,y) x = 7+ 4y}
17) x - 4y = 1
x= 7+ 4y
A) No solution
B) {(x,y)|x - 4y = 1)​

Answer 1

`13.\ (x,y) = (-4,3)`

`14.\ No\ Solution`

`15.\ (x,y) = (-12,-32)`

`16.\ (x,y) = (3.75,6.25)`

`17.\ No\ Solution`

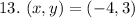
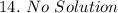
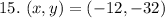
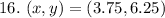
Step-by-step explanation:

13)

`4x + 8y = 8` ---- (1)

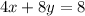`5x + 7y = 1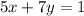` ------ (2)

Multiply equation by 5 and equation 2 by 4

`5(4x + 8y = 8)`

`4(5x + 7y = 1)`

`5 * 4x + 5 * 8y = 5 * 8\\4*5x + 4*7y = 4 *1`

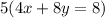
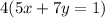
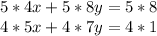
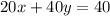`20x + 40y = 40` ------ (3)

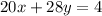`20x + 28y = 4` ------ (4)

Subtract equation 3 from 4

`(20x + 28y = 4) - (20x + 40y = 40)`

`20x - 20x+ 28y -40y= 4-40`

`-12y = -36`

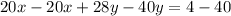
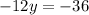
Divide both sides by -12

`(-12y)/(-12) = (-36)/(-12)`

`y = 3`

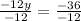
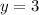
Substitute
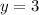`y = 3` in equation 1

`4x + 8y = 8`

`4x + 8(3) = 8`

`4x + 24 = 8`

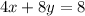
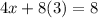
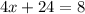
Subtract 24 from both sides

`4x + 24 - 24 = 8 - 24`

`4x = -16`

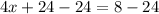
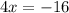
Divide both sides by 4

`(4x)/(4) = (-16)/(4)`

`x = -4`

`Hence\ (x,y) = (-4,3)`

----------------------------------------------

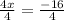
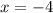
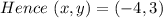
14)

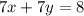`7x + 7y = 8` ---- (1)

`5x + 5y = 10` ----- (2)

Make x the subject of formula in (2)

`5x + 5y = 10`

`5x + 5y - 5y = 10 - 5y`

`5x = 10 - 5y`

`(5x)/(5) = (10 - 5y)/(5)`

`x = (10)/(5) - (5y)/(5)`

`x = 2 - y`

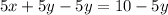
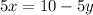
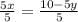
Substitute
`x = 2 - y` in (1)

`7x + 7y = 8`

`7(2-y) + 7y = 8`

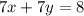
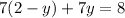
Open bracket

`7*2-7*y + 7y = 8`

`14 -7y + 7y = 8`

`14 \\eq 8`

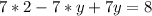
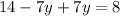
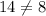
At this point, we conclude that thee system has no solution.

----------------------------------------------

15)

`y = 3x + 4` --- 1

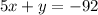`5x + y = -92` ----2

Substitute
`y = 3x + 4` in (2)

`5x + 3x + 4 = -92`

`8x + 4 = -92`

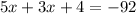
Subtract 4 from both sides

`8x + 4 -4= -92 -4`

`8x = -96`

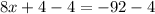
Divide both sides by 8

`(8x)/(8) = (-96)/(8)`

`x = -12`

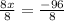
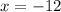
Substitute
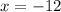`x = -12` in equation 1

`y = 3x + 4`

`y = 3(-12) + 4`

`y = -36 + 4`

`y = -32`

`Hence\ (x,y) = (-12,-32)`

----------------------------------------------

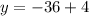
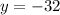
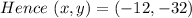
16)

`0.4x + 0.4y = 4`

`0.4x - 0.4y = 1`

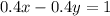
Add both equations

`(0.4x + 0.4y = 4) + (0.4x - 0.4y = 1)`

`0.4x+0.4x + 0.4y-0.4y = 4+1`

`0.8x = 5`

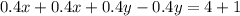
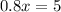
Divide both sides by 0.8

`(0.8x)/(0.8) = (5)/(0.8)`

`x = 6.25`

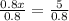
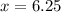
Substitute
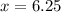`x = 6.25` in (1)

`0.4(6.25) + 0.4y = 4`

`2.5 + 0.4y = 4`

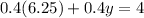
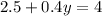
Subtract 2.5 from both sides

`2.5 -2.5 + 0.4y = 4 -2.5`

`0.4y = 1.5`

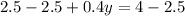
Divide both sides by 0.4

`(0.4y)/(0.4) = (1.5)/(0.4)`

`y = (1.5)/(0.4)`

`y = 3.75`

`Hence,\ (x,y) = (3.75,6.25)`

----------------------------------------------

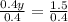
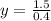
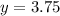
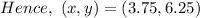
17)

`x - 4y = 1`

`x= 7+ 4y`

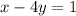
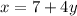
Substitute
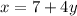`x= 7+ 4y` in (1)

`7 + 4y - 4y = 1`

`7 \\eq 1`

At this point, we conclude that thee system has no solution.