Answer:
z^2/(x^3y)
Explanation:
We assume you want to simplify ...
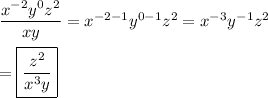
_____
Comment on the given expression
What you have written is ...
(x^-2y^0z^2/x)y = yz^2/x^3
The order of operations requires that division and multiplication be done in the order of appearance, so a/xy means (a/x)y unless you put parentheses on the denominator product. Typesetting the expression lets you use the fraction bar for grouping, so parentheses are not needed when that form is used.