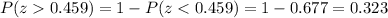Question

The mean number of words per minute (WPM) typed by a speed typist is 135 135 with a variance of 100 100 . What is the probability that the sample mean would be greater than 135.7 135.7 WPM if 43 43 speed typists are randomly selected

Answer 1

`z = (135.7-135)/((10)/(√(43)))= 0.459`

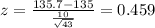
And we can find this probability with this way:

`P(z> 0.459) =1 -P(z<0.459)= 1-0.677= 0.323`

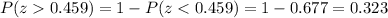
Explanation:

For this case w ehave the following info given:

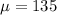`\mu = 135` represent the sample mean

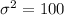`\sigma^2 = 100` represent the sample variance

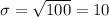`\sigma =√(100)=10` represent the deviation

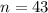`n = 43` represent the sample mean selected

For this case we want to find the following probability:

`P(\bar X >135.7)`

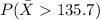
And since the sample size is large enough we can use the following distribution for the sample mean:

`\bar X \sim N(\mu , (\sigma)/(√(n)))`

And we can use the z score formula given by:

`z= (\bar X -\mu)/((\sigma)/(√(n)))`

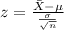
And we can find the z score for the value 135.7 and we got:

`z = (135.7-135)/((10)/(√(43)))= 0.459`

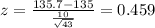
And we can find this probability with this way:

`P(z> 0.459) =1 -P(z<0.459)= 1-0.677= 0.323`