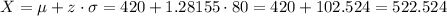Answer:
The minimum score that she must earn to get admitted is 523.
Explanation:
As the scores are normally distributed, we can calculate the probability using the z-score.
The distribution has a mean of 420 and a standard deviation of 80.
We have to calculate the z-score z* that satisfies:
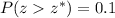
This happens for z*=1.28155.
Then, we can calculate the score as: