Question

A golfer is teeing off on a 130.0 m long par 3 hole. The ball leaves with a velocity of 35.0 m/s at 35.0° to the horizontal. Assuming

that she hits the ball on a direct path to the hole, how far from the hole will the ball land (no bounces or rolls)?

Answer 1

The ball will land 12.5m before that hole.

Step-by-step explanation:

The motion of the golf ball can be considered as a projectile motion, where first its height is 0, then it keeps increasing to a maximum point, after which its height starts decreasing again and hits the ground (height becomes 0 again).

We need to calculate the Range (horizontal distance) of the ball which is given as:

`R=\frac{v_i^2{\cdot}sin2\theta}{g}`

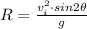
Where

v(i) = initial velocity = 35 m/s

θ = Initial angle = 35°

g = gravitational acceleration = 9.8 m/s

Substitute in the given formula to find Range:

`R=\frac{(35)^2\cdot{sin}(2)(35)}{9.8}\\R=((1225)(0.94))/(9.8)\\R = 117.5~m`

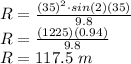
Distance between the hole and position where ball lands = 130m - 117.5m = 12.5m