Question

g A supermarket has three employees who equally packages and weighs produce. Employee A records the correct weight 96% of the time. Employees B and C record the correct weight 95% and 94% of the time, respectively. One customer complains about the incorrect weight recorded on a package he has purchased. What is the probability that the package was weighed by Employee C?

Answer 1

40% probability that the package was weighed by Employee C

Explanation:

Bayes Theorem:

Two events, A and B.

`P(B|A) = (P(B)*P(A|B))/(P(A))`

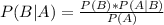
In which P(B|A) is the probability of B happening when A has happened and P(A|B) is the probability of A happening when B has happened.

In this question:

Event A: Incorrect package.

Event B: Weighed by Employee C.

A supermarket has three employees who equally packages and weighs produce.

This means that
`P(B) = (1)/(3)`

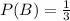
Employee C records the correct weight 94% of the time

So incorrectly 6% of the time, which means that
`P(A|B) = 0.06`

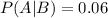
Probability of an incorrect package:

4% of 1/3(A)

5% of 1/3(B)

6% of 1/3(C)

So

`P(A) = (0.04 + 0.05 + 0.06)/(3) = 0.05`

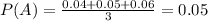
What is the probability that the package was weighed by Employee C?

`P(B|A) = ((1)/(3)*0.06)/(0.05) = 0.4`

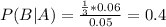
40% probability that the package was weighed by Employee C