Question

Find the value of the linear correlation coefficient r. The paired data below consist of the temperatures on randomly chosen days and the amount a certain kind of plant grew (in millimeters). Temperature 62 76 50 51 71 46 51 44 79 Growth 36 39 50 13 33 33 17 6 16 Question options

Answer 1

n=9
`\sum x = 530, \sum y = 243, \sum xy = 14615, \sum x^2 =32656, \sum y^2 =8245`

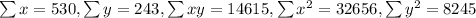
And replacing the info we got:

`r=(9(14615)-(530)(243))/(√([9(32656) -(530)^2][9(8245) -(243)^2]))=0.1955`

So then the correlation coefficient would be r =0.1955

Explanation:

Data given

Temperature (x) 62 76 50 51 71 46 51 44 79

Growth (y) 36 39 50 13 33 33 17 6 16

Solution

The correlation formula is given by:

`r=(n(\sum xy)-(\sum x)(\sum y))/(√([n\sum x^2 -(\sum x)^2][n\sum y^2 -(\sum y)^2]))`

For our case we have the following sums:

n=9
`\sum x = 530, \sum y = 243, \sum xy = 14615, \sum x^2 =32656, \sum y^2 =8245`

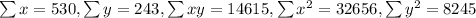
And replacing the info we got:

`r=(9(14615)-(530)(243))/(√([9(32656) -(530)^2][9(8245) -(243)^2]))=0.1955`

So then the correlation coefficient would be r =0.1955