Question

uppose that the length of 20 years worth of baseball games has been investigated, and that it has been found that the average (mean) length of a game is 165 minutes and the standard deviation is 30 minutes. What is the probability that a randomly selected game will last between 120 and 210 minutes

Answer 1

P(120< x < 210) = 0.8664

Explanation:

given data

time length = 20 year

average mean time μ = 165 min

standard deviation σ = 30 min

randomly selected game between = 120 and 210 minute

solution

so here probability between 120 and 210 will be

P(120< x < 210) =
`P((120-165)/(30)< (x-\mu )/(\sigma ) <(210-165)/(30))`

P(120< x < 210) =
`P((-45)/(30)< (x-\mu )/(\sigma ) <(45)/(30))`

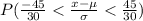
P(120< x < 210) = P(-1.5< Z < 1.5)

P(120< x < 210) = P(Z< 1.5) - P(Z< -1.5)

now we will use here this function in excel function

=NORMSDIST(z)

=NORMSDIST(-1.5)

P(120< x < 210) = 0.9332 - 0.0668

P(120< x < 210) = 0.8664