Question

The axis of symmetry for the graph of the function f(x)=3x2+bx+4 is x equals three-halves. What is the value of b? −18 −9 9 18

Answer 1

b= -9

Explanation:

I just took the quiz and got it right.

Answer 2

b = -9

Explanation:

If we have a function with the form:

`f(x)=ax^2+bx+c`

The axis of symmetry is equal to:

`x = (-b)/(2a)`

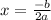
So, for the function
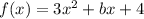`f(x)=3x^2+bx+4`, the value of a is 3 and the value of c is 4. It means that its axis of symmetry is equal to:

`x=(-b)/(2(3))=(-b)/(6)`

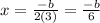
Solving for b, we get that:
`b=-6x`

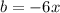
Additionally, the axis of symmetry is
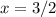`x=3/2`, so replacing x by 3/2, we get that b is equal to:

`b=-6(3/2)\\b=-9`