Answer:
The standard deviation for the income of super shoppers is 76.12.
Explanation:
The formula to compute the standard deviation for the grouped data probability distribution is:
![\sigma=\sqrt{\sum [(x-\mu)^(2)\cdot P(x)]}](https://img.qammunity.org/2021/formulas/mathematics/college/5xle2rod516qdidel5jy0w9bul4j40cnjt.png)
Here,
x = midpoints
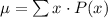
Consider the Excel table attached below.
The mean is:
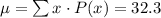
Compute the standard deviation as follows:
![\sigma=\sqrt{\sum [(x-\mu)^(2)\cdot P(x)]}](https://img.qammunity.org/2021/formulas/mathematics/college/5xle2rod516qdidel5jy0w9bul4j40cnjt.png)
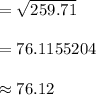
Thus, the standard deviation for the income of super shoppers is 76.12.