Question

Find S20 of the series whose first terms are:
30, 22, 14, 6, ...

Answer 1

- 920

Explanation:

There is a common difference d between consecutive terms in the sequence, that is

d = 22- 30 = 14 - 22 = 6 - 14 = - 8

This indicates the sequence is arithmetic with sum to n terms given by

`S_(n)` =
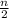`(n)/(2)` [ 2a₁ + (n - 1)d ]

where a₁ is the first term and d the common difference

Here a₁ = 30 and d = - 8 , thus

`S_(20)` =
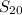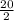`(20)/(2)` [ (2 × 30) + (19 × - 8) ]

= 10(60 - 152)

= 10 × - 92

= - 920