Question

We wish to see if the dial indicating the oven temperature for a certain model of oven is properly calibrated. Four ovens of this model are selected at random. The dial on each is set to 300 °F, and, after one hour, the actual temperature of each is measured. The temperatures measured are 305 °F, 310 °F, 300 °F, and 305 °F. Assuming that the actual temperatures for this model when the dial is set for 300° are Normally distributed with mean μ, we test whether the dial is properly calibrated at 5% of significance level.

Actual Temp: 305, 310, 300, 305

Required:
a. Based on the data, calculate the sample standard deviation and standard error of X bar (round them into two decimal places) Standard Deviation: Standard Error:
b. What is a 95% confidence interval for μ? (upper and lower bound)
c. Provide your test statistic and P-value
d. State your conclusion clearly (statistical conclusion and its interpretation).
e. Even if 5% of significance level looks like default of test, we can use different significance levels as well. If we change the significance level into 10% (= 0.1), how does it affect your conclusion?

Answer 1

a. Standard deviation: 4.082

Standard error: 2.041

b. The 95% confidence interval for the actual temperature is (298.5, 311.5).

Upper bound: 311.5

Lower bound: 298.5

c. Test statistic t=2.45

P-value = 0.092

d. There is no enough evidence to claim that the dial of the oven is not properly calibrated. The actual temperature does not significantly differ from 300 °F.

e. If we use a significance level of 10% (a less rigorous test, in which the null hypothesis is rejected with with less requirements), the conclusion changes and now there is enough evidence to claim that the dial is not properly calibrated.

This happens because now the P-value (0.092) is smaller than the significance level (0.10), given statististical evidence for the claim.

Explanation:

The mean and standard deviation of the sample are:

`M=(1)/(4)\sum_(i=1)^(4)(305+310+300+305)\\\\\\ M=(1220)/(4)=305`

`s=\sqrt{(1)/((n-1))\sum_(i=1)^(4)(x_i-M)^2}\\\\\\s=\sqrt{(1)/(3)\cdot [(305-(305))^2+(310-(305))^2+(300-(305))^2+(305-(305))^2]}\\\\\\ s=\sqrt{(1)/(3)\cdot [(0)+(25)+(25)+(0)]}\\\\\\ s=\sqrt{(50)/(3)}=√(16.667)\\\\\\s=4.082`

We have to calculate a 95% confidence interval for the mean.

The population standard deviation is not known, so we have to estimate it from the sample standard deviation and use a t-students distribution to calculate the critical value.

The sample mean is M=305.

The sample size is N=4.

When σ is not known, s divided by the square root of N is used as an estimate of σM (standard error):

`s_M=(s)/(√(N))=(4.082)/(√(4))=(4.082)/(2)=2.041`

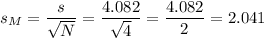
The degrees of freedom for this sample size are:

`df=n-1=4-1=3`

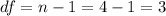
The t-value for a 95% confidence interval and 3 degrees of freedom is t=3.18.

The margin of error (MOE) can be calculated as:

`MOE=t\cdot s_M=3.18 \cdot 2.041=6.5`

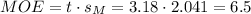
Then, the lower and upper bounds of the confidence interval are:

`LL=M-t \cdot s_M = 305-6.5=298.5\\\\UL=M+t \cdot s_M = 305+6.5=311.5`

The 95% confidence interval for the actual temperature is (298.5, 311.5).

This is a hypothesis test for the population mean.

The claim is that the actual temperature of the oven when the dial is at 300 °F does not significantly differ from 300 °F.

Then, the null and alternative hypothesis are:

`H_0: \mu=300\\\\H_a:\mu\\eq 300`

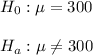
The significance level is 0.05.

The sample has a size n=4.

The sample mean is M=305.

As the standard deviation of the population is not known, we estimate it with the sample standard deviation, that has a value of s=4.028.

The estimated standard error of the mean is computed using the formula:

`s_M=(s)/(√(n))=(4.082)/(√(4))=2.041`

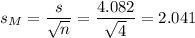
Then, we can calculate the t-statistic as:

`t=(M-\mu)/(s/√(n))=(305-300)/(2.041)=(5)/(2.041)=2.45`

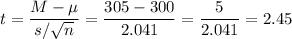
The degrees of freedom for this sample size are:

`df=n-1=4-1=3`

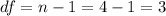
This test is a two-tailed test, with 3 degrees of freedom and t=2.45, so the P-value for this test is calculated as (using a t-table):

`\text{P-value}=2\cdot P(t>2.45)=0.092`

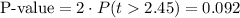
As the P-value (0.092) is bigger than the significance level (0.05), the effect is not significant.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that the actual temperature of the oven when the dial is at 300 °F does not significantly differ from 300 °F.

If the significance level is 10%, the P-value (0.092) is smaller than the significance level (0.1) and the effect is significant.

The null hypothesis is rejected.

There is enough evidence to support the claim that the actual temperature of the oven when the dial is at 300 °C does not significantly differ from 300 °C.