Question

Two machines used to fill soft drink containers are being compared. The number of containers filled each minute is counted for 60 minutes for each machine. During the 60 minutes, machine 1 filled an average of 73.8 cans per minute with a standard deviation of 5.2 cans per minute, and machine 2 filled an avaerage of 76.1 cans per minute with a standard deviation of 4.1 cans per minute.

Required:
a. If the counts are made each minute for 60 consecutiveminutes, what assumption necessary to the validity of a hypothesistest may be violated?
b. Assuming that all necessary assumptions are met, perform a hypothesis test. Can you conclude that machine 2 is faster than machine 1?

Answer 1

The assumption necessary to the validity of a hypothesis test that may be violated in this case is the assumption of independence. A two-sample t-test can be used to perform a hypothesis test to compare the average number of containers filled by the two machines. The conclusion about the speed of machine 2 cannot be made without performing the hypothesis test.

Step-by-step explanation:

a. One assumption necessary to the validity of a hypothesis test that may be violated in this case is the assumption of independence. If the counts of containers filled by each machine are not independent of each other, it can affect the validity of the hypothesis test.

b. To perform a hypothesis test, we can use a two-sample t-test. The null hypothesis would be that there is no significant difference between the average number of containers filled by machine 1 and machine 2. We can calculate the test statistic and compare it to the critical value to make a conclusion.

Based on the provided information, we cannot conclude that machine 2 is faster than machine 1 without performing the hypothesis test.

Answer 2

The calculated value |t| = | - 2.6932 | = 2.6932 > 1.9803 at 0.05 level of significance

Alternative hypothesis is accepted

The average of machine two is faster than machine one

Step-by-step explanation:

Step(i):-

Given sample size n₁ = n₂ = 60 minutes

The average of first sample 'x⁻₁ = 73.8

The standard deviation of the first sample 'S₁ ' = 5.2 cans per minute

The average of second sample 'x⁻₂ = 76.1

The standard deviation of the second sample 'S₂ ' = 4.1 cans per minute

step(ii):-

Null Hypothesis : H₀: 'x⁻₁ = 'x⁻₂

Alternative Hypothesis : H₁ : 'x⁻₁ > 'x⁻₂

Test statistic

`t = \frac{x^(-) _(1) - x^(-) _(2) }{\sqrt{(S^(2) _(1) )/(n_(1) ) +(S^(2) _(2) )/(n_(2) ) } }`

`t = \frac{73.8 - 76.1 }{\sqrt{((5.2)^(2) )/(60 ) +((4.1)^(2) )/(60 ) } }`

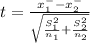
t = - 2.6932

|t| = | - 2.6932 | = 2.6932

Step(iii):-

Degrees of freedom

ν = n₁ + n₂ -2 = 60 +60 -2 = 118

t₀.₀₅ = 1.9803

The calculated value |t| = | - 2.6932 | = 2.6932 > 1.9803 at 0.05 level of significance

Final answer:-

Null hypothesis is rejected at 0.05 level of significance

Alternative hypothesis is accepted

The average of machine two is faster than the average of machine one