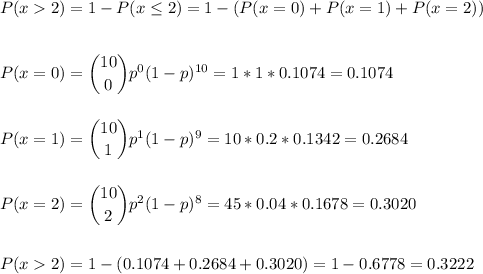Answer:
A. P=0.4013
B. P=0.9884
C. P=0.3222
Explanation:
We can model this with a binomial random variable.
The sample size is n=10.
The probability is p=0.05 for scrap and p=0.05+0.15=0.20 for degraded or scrap.
The probability of having k scrap gears in the sample is:
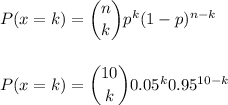
The probability that one or more is scrap can be calculated as 100% less the probability that no one is scrap:
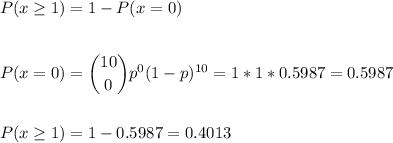
The probability that 8 or more are not scrap is equal to the probability of having 2 or less that are scrap:
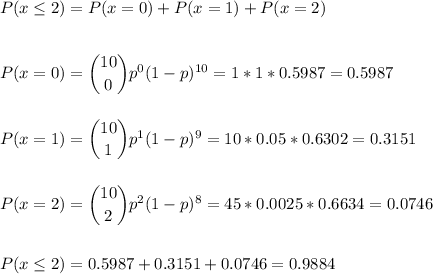
The probability that more than two are degraded or scrap (p=0.2) is calculated as: