Question

According to statcounter, Google Chrome browser controls 62.8% of the market share worldwide. A random sample of 70 users was selected. What is the probability that 35 or more from this sample used Google Chrome as their browser

Answer 1

The probability that 35 or more users from the sample of 70 used G o o g l e Chrome as their browser is approximately 0.972.

In order to find the probability that 35 or more users from the sample of 70 used G o o g l e Chrome as their browser, we can use the binomial distribution formula.

The formula is: P(X >= k) = 1 - P(X < k-1), where P(X >= k) is the probability of getting at least k successes (in this case, 35 or more users using G o o g l e Chrome).

Using the formula and the given information that G o o g l e Chrome has a market share of 62.8%, we can calculate the probability.

P(X >= 35) = 1 - P(X < 34) = 1 - binomcdf(70, 0.628, 34) = 0.972

Therefore, the probability that 35 or more users from the sample used G o o g l e Chrome as their browser is approximately 0.972.

Answer 2

The probability that 35 or more from this sample used Google Chrome as their browser is 0.9838.

Explanation:

We are given that according to Statcounter, the Google Chrome browser controls 62.8% of the market share worldwide.

A random sample of 70 users was selected.

Let
`\hat p` = sample proportion of users who used Google Chrome as their browser.

The z-score probability distribution for the sample proportion is given by;

Z =
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n) } }` ~ N(0,1)

where,
`\hat p` = sample proportion =
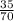`(35)/(70)` = 0.50

p = population proportion = 62.8%

n = sample of users = 70

Now, the probability that 35 or more from this sample used Google Chrome as their browser is given by = P(
`\hat p`
`\geq` 0.50)

P(
`\hat p`
`\geq` 0.50) = P(
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n) } }`
`\geq`
`\frac{0.50-0.628}{\sqrt{(0.50(1-0.50))/(70) } }` ) = P(Z
`\geq` -2.14)

= P(Z
`\leq` 2.14) = 0.9838

The above probability is calculated by looking at the value of x = 2.14 in the z table which has an area of 0.9838.