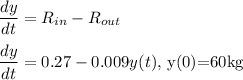Answer:
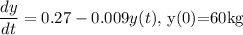
Explanation:
Volume of water in the tank = 1000 L
Let y(t) denote the amount of salt in the tank at any time t.
Initially, the tank contains 60 kg of salt, therefore:
y(0)=60 kg
Rate In
A solution of concentration 0.03 kg of salt per liter enters a tank at the rate 9 L/min.
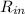 =(concentration of salt in inflow)(input rate of solution)
=(concentration of salt in inflow)(input rate of solution)
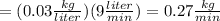
Rate Out
The solution is mixed and drains from the tank at the same rate.
Concentration,
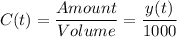
 =(concentration of salt in outflow)(output rate of solution)
=(concentration of salt in outflow)(output rate of solution)
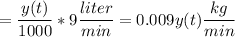
Therefore, the differential equation for the amount of Salt in the Tank at any time t: