Answer:
The expression as a sum of terms is
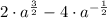 .
.
Explanation:
The expression to be rewritten has the following form:

A polynomial as a sum of terms consists in a sum of terms of the form
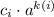 , where
, where
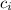 and
and
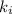 are the i-th coefficient and exponent. The expression has to be expanded to create the sum of terms:
are the i-th coefficient and exponent. The expression has to be expanded to create the sum of terms:
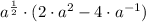
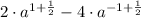
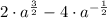
The expression as a sum of terms is
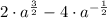 .
.