Question

Some have argued that throwing darts at the stock pages to decide which companies to invest in could be a successful​ stock-picking strategy. Suppose a researcher decides to test this theory and randomly chooses 250 companies to invest in. After 1​ year, 135 of the companies were considered​ winners; that​ is, they outperformed other companies in the same investment class. To assess whether the​ dart-picking strategy resulted in a majority of​ winners, the researcher tested Upper H 0​: pequals0.5 versus Upper H 1​: pgreater than0.5 and obtained a​ P-value of 0.1030. Explain what this​ P-value means and write a conclusion for the researcher.​

Answer 1

The calculated value Z = 1.2903 < 1.96 at 0.05 level of significance

Null hypothesis is accepted at 0.05 level of significance

The population proportion is equal to 0.5

Explanation:

Step (i):-

Given random sample size ' n' = 250

Sample proportion 'p'

`p= (x)/(n) = (135)/(250) = 0.54`

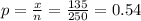
Given Population proportion P = 0.5

Q = 1-P = 1-0.5 =0.5

Null Hypothesis : H₀ : P = 0.5

Alternative Hypothesis : H₁ : P≥ 0.5

Step(ii):-

Test statistic

`Z = \frac{p - P}{\sqrt{(PQ)/(n) } }`

`Z = \frac{0.54-0.5}{\sqrt{(0.5 X 0.5)/(250) } }`

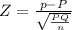
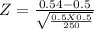
Z = 1.2903

Level of significance α = 0.05

Z₀.₀₅ = 1.96

The calculated value Z = 1.2903 < 1.96 at 0.05 level of significance

Null hypothesis is accepted at 0.05 level of significance

Step(iii):-

P- value

The probability of test statistic

P(Z > 1.2903) = 0.5 - A ( 1.2903)

= 0.5 - 0.4015

= 0.0985≅ 0.10

i) P- value =0.10 > α = 0.05

null hypothesis is accepted

Conclusion:-

The population proportion is equal to 0.5