Answer:
 (b)
(b)
The critical value for 80% of confidence interval now can be founded using the normal distribution the significance level would be 20% and the critical value
 , replacing into formula (b) we got:
, replacing into formula (b) we got:
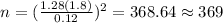
So the answer for this case would be n=369 rounded up to the nearest integer
Explanation:
We know the following info given:
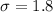 represent the standard deviation
represent the standard deviation
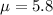 the true mean that she believes
the true mean that she believes
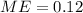 represent the margin of error
represent the margin of error
The margin of error is given by this formula:
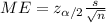 (a)
(a)
And on this case we have that ME =+0.12 and we are interested in order to find the value of n, if we solve n from equation (a) we got:
 (b)
(b)
The critical value for 80% of confidence interval now can be founded using the normal distribution the significance level would be 20% and the critical value
 , replacing into formula (b) we got:
, replacing into formula (b) we got:
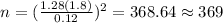
So the answer for this case would be n=369 rounded up to the nearest integer