Question

Circle C is shown. Line segments E C and C D are radii. Lines are drawn from points E and D to point F on the other side of the circle. Arc E D measures 95 degrees. What is the measure of angle EFD? 37.5° 45° 47.5° 55°

Answer 1

its 47.5

Explanation:

i got it right on edge

Answer 2

(C)
`47.5^\circ`

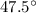
Explanation:

In the circle, the measure of arc ED is the measure of an angle subtended by arc ED at the centre.

Angle EFD on the other hand is an angle subtended by arc ED on the circumference.

We have a circle's theorem that states:

The angle subtended by the arc at the centre is twice the angle subtended by the same arc at the circumference, therefore:

`m\angle ED=2 * m\angle EFD\\95^\circ=2 * m\angle EFD\\m\angle EFD=95 / 2\\m\angle EFD=47.5^\circ`