Answer:
a) 1.46%.
b) 92.33%.
c) 0.32%.
d) 50%
Explanation:
When the distribution is normal, we use the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the zscore of a measure X is given by:
, the zscore of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
In this question, we have that:

a) catch a dragonfly in less than 18 seconds;
This is the pvalue of Z when X = 18. So

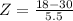

 has a pvalue of 0.0146
has a pvalue of 0.0146
So the percentage of shrews is 1.46%.
b) catch a dragonfly in between 22 and 45 seconds;
This is the pvalue of Z when X = 45 subtracted by the pvalue of Z when X = 22.
X = 45

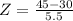
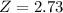
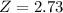 has a pvalue of 0.9968
has a pvalue of 0.9968
X = 22

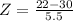

 has a pvalue of 0.0735
has a pvalue of 0.0735
0.9968 - 0.0735 = 0.9233
So the answer is 92.33%.
c) take longer than 45 seconds to catch a dragonfly?
From b), when X = 45, Z = 2.73 has a pvalue of 0.9968
1 - 0.9968 = 0.0032
So the answer for this item is 0.32%.
d) take less than 30 seconds to catch its prey;
This is the pvalue of Z when X = 30.

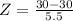

 has a pvalue of 0.5
has a pvalue of 0.5
So the answer for d) is 50%.