Question

Write an exponential equation in the form y = abx whose graph passes through points (−3, 24) and (−2, 12). y = 3x y = 3(0.5)x y = 0.5(3)x y = −3(24)x

Answer 1

Option B.

Explanation:

The given exponential equation is

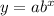`y=ab^x` ...(1)

It is given that the graph of above equation passes through points (−3, 24) and (−2, 12).

The graph passes through the point (-3,24), so substitute x=-3 and y=24 in equation (1).

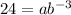`24=ab^(-3)` ...(2)

The graph passes through the point (-2,12), so substitute x=-2 and y=12 in equation (1).

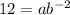`12=ab^(-2)` ...(3)

Divide equation (3) by equation (2).

`(12)/(24)=(ab^(-2))/(ab^(-3))`

`0.5=b`

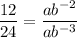
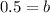
Substitute b=0.5 in equation (2).

`24=a(0.5)^(-3)`

`24=(a)/((0.5)^(3))`

`24=(a)/(0.125)`

`24* 0.125=a`

`3=a`

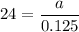
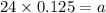
Substitute a=3 and b=0.5 in equation (1).

`y=3(0.5)^x`

Therefore, the correct option is B.