Answer:
The final temperature and pressure in the insulated rigid tank are
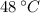 and
and
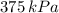 .
.
Step-by-step explanation:
An ideal gas is represented by the following model:
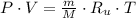
Where:
 - Pressure, measured in kilopascals.
- Pressure, measured in kilopascals.
 - Volume, measured in cubic meters.
- Volume, measured in cubic meters.
 - Mass of the ideal gas, measured in kilograms.
- Mass of the ideal gas, measured in kilograms.
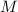 - Molar mass, measured in kilograms per kilomole.
- Molar mass, measured in kilograms per kilomole.
 - Temperature, measured in Kelvin.
- Temperature, measured in Kelvin.
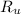 - Universal constant of ideal gases, equal to
- Universal constant of ideal gases, equal to
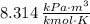
As tank is rigid and insulated, it means that no volume deformations in tank, heat and mass interactions with surroundings occur during expansion process. Hence, final pressure is less that initial one, volume is doubled (due to equal partitioning) and temperature remains constant. Hence, the following relationship can be derived from model for ideal gases:
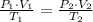
Now, final pressure is cleared:
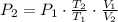
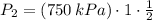
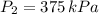
The final temperature and pressure in the insulated rigid tank are
 and
and
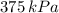 .
.