Question

5. Germanium forms a substitutional solid solution with silicon. Compute the number of germanium atoms per cubic centimeter for a germanium-silicon alloy that contains 15 wt% Ge and 85 wt% Si. The densities of pure germanium and silicon are 5.32 and 2.33 g/cm3, respectively. Atomic weight of Ge is 72.64 g/mol

Answer 1

There are
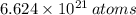`6.624 * 10^(21)\,atoms` of Germanium in a germanium-silicon alloy that contains 15 wt% Ge and 85 wt% Si.

Step-by-step explanation:

The masses of silicon and germanium contained in a cubic centimeter of the germanium-silicon alloy by apply the concepts of mass (
`m`), density (
`\rho`) and volume (
`V`), as well as the mass-mass proportion of Germanium (
`x`):

`m_(Ge) = x \cdot \rho_(Ge)\cdot V_(sample)`

`m_(Ge) = 0.15\cdot \left(5.32\,(g)/(cm^(3)) \right)\cdot (1\,cm^(3))`

`m_(Ge) = 0.798\,g`

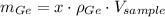
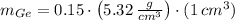
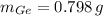
The amount of moles of Germanium is obtained after dividing previous outcome by its atomic weight. That is to say:

`n = (m_(Ge))/(M_(Ge))`

`n = (0.798\,g)/(72.64\,(g)/(mol) )`

`n = 0.011\,mol`

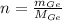
There are 0.011 moles in a cubic centimeter of the germanium-silicon alloy. According to the Law of Avogadro, there are
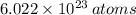`6.022 * 10^(23)\,atoms` in a mole of Germanium. The quantity of atoms in a cubic centimeter is therefore found by simple rule of three:

`y = (0.011\,mol)/(1\,mol)* \left(6.022* 10^(23)\,(atoms)/(mole) \right)`

`y = 6.624 * 10^(21)\,atoms`

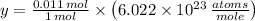
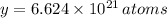
There are
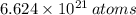`6.624 * 10^(21)\,atoms` of Germanium in a germanium-silicon alloy that contains 15 wt% Ge and 85 wt% Si.