Answer:
a) a = 5.03x10¹³ m/s²
b)
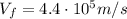
Explanation:
a) The acceleration of the positron can be found as follows:
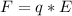 (1)
(1)
Also,
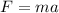 (2)
(2)
By entering equation (1) into (2), we have:
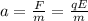
Where:
F: is the electric force
m: is the particle's mass = 9.1x10⁻³¹ kg
q: is the charge of the positron = 1.6x10⁻¹⁹ C
E: is the electric field = 286 N/C
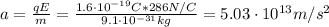
b) The positron's speed can be calculated using the following equation:

Where:
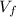 : is the final speed =?
: is the final speed =?
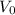 : is the initial speed =0
: is the initial speed =0
t: is the time = 8.70x10⁻⁹ s
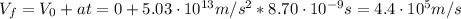
I hope it helps you!