Question

A bank is investigating ways to entice customers to charge more on their credit cards. (Banks earn a fee from the merchant on each purchase, and hope to collect interest from the customers, as well.) A bank selects a random group of customers who are told their "cash back" will increase from 1% to 2% for all charges above a certain dollar amount each month. Of the 500 customers who were told the increase applied to charges above $1000 each month, the average increase in spending was $527 with a standard deviation of $225. Of the 500 customers who were told the increase applied to charges above $2000 each month, the average increase in spending was $439 with a standard deviation of $189. A level C = 95% confidence interval for \mu_1\:-\:\mu_2μ 1 − μ 2 is approximated by Group of answer choices (62.2, 113.8) (86.2, 120.5) (10.3, 23.8) (55.6, 67.8)

Answer 1

`CI = (\bar{x_(1) } - \bar{x_(2)} ) \pm MoE\\\\`

`CI = (527 - 439) \pm 25.75\\\\CI = 88 \pm 25.75\\\\CI = 88 - 25.75 \:\: and \:\: 88 + 25.75\\\\CI = (62.2 \: ,\: 113.8 )`

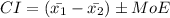
The correct answer choice is a. (62.2, 113.8)

Explanation:

Of the 500 customers who were told the increase applied to charges above $1000 each month, the average increase in spending was $527 with a standard deviation of $225.

Sample size = n₁ = 500

Sample mean = x₁ = $527

Standard deviation = s₁ = $225

Of the 500 customers who were told the increase applied to charges above $2000 each month, the average increase in spending was $439 with a standard deviation of $189

Sample size = n₂ = 500

Sample mean = x₂ = $439

Standard deviation = s₂ = $189

We are asked to find the 95% confidence interval for the difference between two means.

The given group of answer choices are

a. (62.2, 113.8)

b. (86.2, 120.5)

c. (10.3, 23.8)

d. (55.6, 67.8)

The confidence interval for the difference between two means is given by

`CI = (\bar{x_(1) } - \bar{x_(2)} ) \pm MoE\\\\`

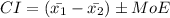
Where
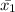`\bar{x_(1) }` and
`\bar{x_(2) }` are the given sample means and margin of error is given by

`$ MoE = z_(\alpha/2) \cdot \sqrt{(s_(1)^2)/(n_1) + (s_(2)^2)/(n_2)} $`

The z-score corresponding to 95% confidence level is given by

Significance level = α = 1 - 0.95 = 0.05/2 = 0.025

From the z-table at α = 0.025 the z-score is 1.96

`$ MoE = 1.96 \cdot \sqrt{(225^2)/(500) + (189^2)/(500)} $`

`MoE = 1.96 \cdot 13.14`

`MoE = 25.75`

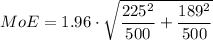
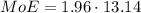
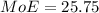
Finally,

`CI = (\bar{x_(1) } - \bar{x_(2)} ) \pm MoE\\\\`

`CI = (527 - 439) \pm 25.75\\\\CI = 88 \pm 25.75\\\\CI = 88 - 25.75 \:\: and \:\: 88 + 25.75\\\\CI = (62.2 \: ,\: 113.8 )`

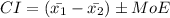
Therefore, the correct answer choice is a. (62.2, 113.8)

How to use z-table?

In the z-table find the probability of 0.025

Note down the value of that row, it would be 1.9.

Note down the value of that column, it would be 0.06.

Add the two numbers together.

The z-score is 1.9 + 0.06 = 1.96