Question

A statistician wants to determine if there is a difference in the fuel efficiency of cars between model years. To do this, he selects random makes and models of cars and compares the fuel efficiency in miles per gallon of the current model year and the previous model year. Suppose that data were collected for a random sample of 9 cars, where each difference is calculated by subtracting the fuel efficiency in miles per gallon of the previous model year from the fuel efficiency in miles per gallon of the current model year. Assume that the fuel efficiencies are normally distributed. The statistician uses the alternative hypothesis Ha:μd≠0. Using a test statistic of t≈6.163, which has 8 degrees of freedom, determine the range that contains the p-value.

Answer 1

P-value = 0.00028

Explanation:

The statician performs an hypothesis test for the mean difference.

As the alternative hypothesis is Ha: μd ≠ 0, we know that it is a two-tailed test.

The test statistic is t=6.163.

For a two-tailed test and 8 degrees of freedom, this corresponds to a P-value of:

`\text{P-value}=2\cdot P(t>6.163)=0.00028`

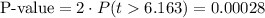
This P-value is smaller enough to reject the null hypothesis at almost any significance level.