Answer:
The table that illustrates this equation is table A:
1 -6 0 up (0,0)
Explanation:
The values of the parameters a, b, and c have to agree with the values for the general quadratic equation in standard form:
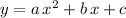
compared to:
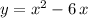
So the coefficient "a" of the quadratic term in our case is: "1"
the coefficient "b" of the linear term is : "-6"
the coefficient "c" for the constant term s : "0" (zero)
since the coefficient "a" is a positive number, we know that the parabola's branches must be opening "UP".
The y intercept can be found by evaluating the expression for x = 0:
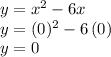
Therefore the y-intercept is at (0, 0)
These results agree with those of Table "A"