Answer:
7:6
Explanation:
The odds that Romance will win are listed as 2 : 3
We can rewrite this as:
The probability that Romance will win

The odds that Downhill will win are 1 : 2.
The probability that Downhill will win
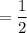
Therefore:
The probability that either Romance or Downhill wins
=P(Romance Wins)+P(Downhill wins)
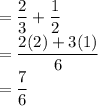
Therefore. the odds that should be given for the event that either Romance or Downhill wins is: 7:6