Answer:
A. xmax = 131.49 m
B. t = 8.74 s
C. ymax = 220.33 m
Step-by-step explanation:
A. In order to find the horizontal distance which cannon travels you first calculate the flight time. The flight time can be calculated by using the following formula:
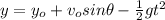 (1)
(1)
yo: height from the projectile is fired = 200m
vo: initial velocity of the projectile = 25m/s
g: gravitational acceleration = 9.8 m/s^2
θ: angle between the direction of the initial motion of the ball and the horizontal = 53°
t: time
You need the value of t when the projectile hits the ground. Then, in th equation (1) you make y = 0m.
When you replace the values of all parameters in the equation (1), you obtain the following quadratic formula:
 (2)
(2)
You use the quadratic formula to obtain the value of t:

You use the positive value because it has physical meaning.
Now, you can calculate the horizontal range of the projectile by using the following formula:
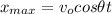
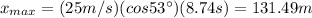
The cannon ball travels a horizontal distance of 131.49 m
B. The cannon ball reaches the canon for t = 8.74s
C. The maximum height is obtained by using the following formula:
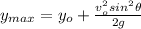 (3)
(3)
By replacing in the equation (3) the values of all parameters you obtain:
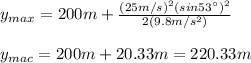
The maximum height reached by the cannon ball is 220.33m