Question

Write the equation of the line parallel to y+4= 1/4(x+5) and passing through the point (8, 20). Write in the format y = mx + b

Answer 1

`y=0.25x+18`

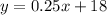
Explanation:

So first we take the equation we are given and write it in slope-intercept form (y = mx + b):

`y+4= (1)/(4) (x+5)\\\\y+4=0.25x +1.25\\\\y=0.25x-2.75`

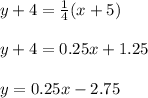
Now we know parallel lines have the same slope, so the line we are looking for has a slope of 0.25.

so we can start to set up our equation:

`y=0.25x+b`

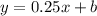
and then substitue in the point (8,20) to find the y-intercept.

`20=0.25(8)+b\\20=2+b\\b=18`

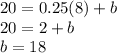
So now we have our equation:

`y=0.25x+18`

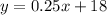
Hope this helps!