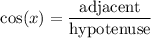Question

The value of cos X is
40/41
42/41
9/41
35/41

Answer 1

I think we're probably talking about a right triangle. So just find the adjacent side and the hypotenuse.

Explanation:

Once we are considering
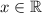`x \in \mathbb{R}`, the range of
`\text{cos}(x)` is
`[1, -1]`. Therefore,
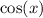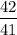`$(42)/(41) $` is not true.

In this exercise, we may have

`$\text{cos}(x)=(x)/(r) =(x)/(√(x^2 +y^2) ) $`

Considering the trigonometric functions beyond the unit circle.

Or considering the definition in the right triangle:

`$\text{cos}(x)=\frac{\text{adjacent}}{\text{hypotenuse}} $`