Question

A sample of 500 nursing applications included 60

from men. Find the 90% confielence interval
for the
true proportion of men who applied to the nursing
program.​

Answer 1

90% confidence interval for the true proportion of men who applied to the nursing program.​

(0.09674 ,0.14326)

Explanation:

Explanation:-

Given sample size 'n' = 500

sample proportion

`p = (x)/(n) = (60)/(500) = 0.12`

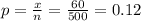
Level of significance ∝= 0.90 or 0.10

90% confidence interval for the true proportion of men who applied to the nursing program.​

`(p - Z_{(0.10)/(2) } \sqrt{(p(1-p))/(n) } , p + Z_{(0.10)/(2) } \sqrt{(p(1-p))/(n) })`

`(p - Z_(0.05 ) \sqrt{(p(1-p))/(n) } , p + Z_(0.05 ) \sqrt{(p(1-p))/(n) })`

`(0.12 - 1.645 \sqrt{(0.12(1-0.12))/(500) } , 0.12 + 1.645 \sqrt{(0.12(1-0.12))/(500) })`

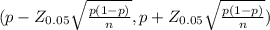
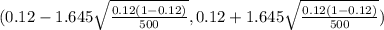
On calculation , we get

( 0.12 - 0.02326 , 0.12 + 0.02326)

(0.09674 ,0.14326)

Final answer:-

90% confidence interval for the true proportion of men who applied to the nursing program.​

(0.09674 ,0.14326)