Answer:
Explanation:
Given:
Isolate the term of y from one side of the equation.
(3y+2)(y-1)
Use the foil method.
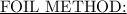
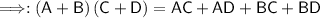
(3y+2)(y-1)=3yy+3y(-1)+2y+2(-1)
Solve.

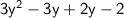
Add or subtract the numbers from left to right.
Solutions:
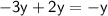
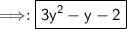
- Therefore, the correct answer is 3y²-y-2.
I hope this helps. Let me know if you have any questions.