Question

A company that manufactures video cameras produces a basic model and a deluxe model. Over the past year, 41% of the cameras sold have been of the basic model. Of those buying the basic model, 31% purchase an extended warranty, whereas 48% of all deluxe purchasers do so. If you learn that a randomly selected purchaser has an extended warranty, how likely is it that he or she has a basic model

Answer 1

`75.6\%`

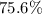
Explanation:

Let B be the event of buying a basic model.

Given that P(B) = 41%

Let D be the event of buying a basic model.

Given that P(D) = 1 - 41% = 59%

Let E be the event of extended warranty.

Given that:

P(E
`\cap` B) = 31% and

P(E
`\cap` D) = 48%

P(E) = P(E
`\cap` B)
`*` P(B) + P(E
`\cap` D)
`*` P(D)

P(E) = 31%
`*` 41% + 48%
`*` 59% = 0.4103

To find: P(B/E)

Formula:

`P(B/E) = (P(E \cap B))/(P(E))`

`\Rightarrow (0.31)/(0.41)\\\Rightarrow 0.756\\\Rightarrow 75.6\%`

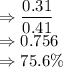
So, the correct answer is
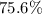`75.6\%`.