Question

sarah can complete a project in 90 minutes and her sister betty can complete it in 120 minutes if they both work on the project at the same time how long will it take them to complete the project

Answer 1

It will take them approximately 51.43 minutes to complete the project together

Explanation:

This is what is called a "shared job" problem.

The best way to work on them is to start by finding the "portion" of the job done by each of the people in the unit of time.

So, for example, Sarah completes the project in 90 minutes, so in the unit of time (that is 1 minute) she completed 1/90 of the total project

Betty completes the project in 120 minutes, so in the unit of time (1 minute) she completes 1/120 of the total project.

We don't know how long it would take for them to complete the project when working together, so we call that time "x" (our unknown).

Now, when they work together completing the entire job in x minutes, in the unit of time they would have done 1/x of the total project.

In the unite of time, the fraction of the job done together (1/x) should equal the fraction of the job done by Sarah (1/90) plus the fraction of the job done by Betty. This in mathematical form becomes:

`(1)/(x) =(1)/(90) +(1)/(120)\\(1)/(x) =(4)/(360) +(3)/(360)\\(1)/(x) =(7)/(360) \\x=(360)/(7) \\x=51.43\,\,min`

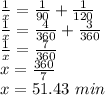
So it will take them approximately 51.43 minutes to complete the project together.