Question

A group of four components is known to contain two defectives. An inspector tests the components one at a time until the two defectives are located. Once she locates the two defectives, she stops testing, but the second defective is tested to ensure accuracy. Let Y denote the number of the test on which the second defective is found. Find the probability distribution for Y. (Enter your probabilities as fractions.)

Answer 1

Explanation:

Let D stand for a defective unit,

G for a good unit.

Y can only be 2, 3, or 4.

P(Y=2):

`=(2)/(4) *(1)/(3) =(1)/(6)`

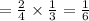
Need to have D, D. There are 2 Ds of 4 total to start, then presuming the first was a D, there is 1 D of 3 total for the second choice.

P(Y=3):

Need to have D,G,D or G,D,D

`=(2)/(4) *(2)/(3) * (1)/(2) +(2)/(4)* (2)/(3)* (1)/(2) \\\\=(1)/(6)+(1)/(6)\\\\=(1)/(3)`

`P(Y=4) = 1 - (P(Y=2) + P(Y=3) \\\\= 1 - (1)/(6) - (1)/(3) \\\\= (1)/(2)`

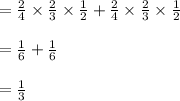
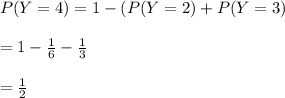
So:

P(Y=2) = 1/6

P(Y=3) = 1/3

P(Y=4) = 1/2

P(Y=n) = 0 for all other values of n