Question

In a survey of a group of​ men, the heights in the​ 20-29 age group were normally​ distributed, with a mean of 69.9 inches and a standard deviation of 4.0 inches. A study participant is randomly selected. Complete parts​ (a) through​ (d) below. ​(a) Find the probability that a study participant has a height that is less than 65 inches. The probability that the study participant selected at random is less than 65 inches tall is nothing. ​(Round to four decimal places as​ needed.)

Answer 1

The probability that a study participant has a height that is less than 65 inches is 0.1103.

Explanation:

We are given that the heights in the​ 20-29 age group were normally​ distributed, with a mean of 69.9 inches and a standard deviation of 4.0 inches.

A study participant is randomly selected.

Let X = heights in the​ 20-29 age group.

So, X ~ Normal(
`(\mu=69.9,\sigma^(2) =4.0^(2)`)

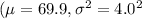The z-score probability distribution for the normal distribution is given by;

Z =
`(X-\mu)/(\sigma)` ~ N(0,1)

where,
`\mu` = mean height = 69.9 inches

`\sigma` = standard deviation = 4.0 inches

Now, the probability that a study participant has a height that is less than 65 inches is given by = P(X < 65 inches)

P(X < 65 inches) = P(
`(X-\mu)/(\sigma)` <
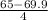`(65-69.9)/(4)` ) = P(Z < -1.225) = P(Z
`\leq` 1.225)

= 1 - 0.8897 = 0.1103

The above probability is calculated by looking at the value of x = 1.225 in the z table which lies between x = 1.22 and x = 1.23 which has an area of 0.88877 and 0.89065 respectively.