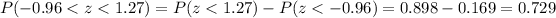Question

Drivers who are members of the teamsters Union earn an average of $17.15 per hour (U.S. News & World Report). Assume that available data indicate wages are normally distributed with a standard deviation of $2.25. 1) What is the probability that wages are between $15.00 and $20.00 per hours?

Answer 1

`P(15<X<20)=P((15-\mu)/(\sigma)<(X-\mu)/(\sigma)<(20-\mu)/(\sigma))=P((15-17.15)/(2.25)<Z<(20-17.15)/(2.25))=P(-0.96<z<1.27)`

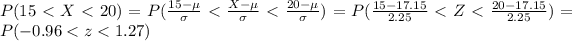
And we can find the probability with this difference and using the normal standard table:

`P(-0.96<z<1.27)=P(z<1.27)-P(z<-0.96)= 0.898-0.169 = 0.729`

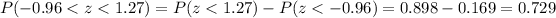
Explanation:

Let X the random variable that represent the wages, and for this case we know the distribution for X is given by:

`X \sim N(17.15,2.25)`

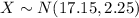
Where
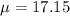`\mu=17.15` and
`\sigma=2.25`

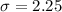
We want to find this probability:

`P(15<X<20)`

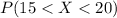
And we can use the z score formula given by:

`z=(x-\mu)/(\sigma)`

Using this formula we have:

`P(15<X<20)=P((15-\mu)/(\sigma)<(X-\mu)/(\sigma)<(20-\mu)/(\sigma))=P((15-17.15)/(2.25)<Z<(20-17.15)/(2.25))=P(-0.96<z<1.27)`

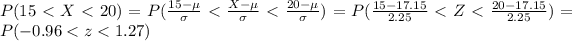
And we can find the probability with this difference and using the normal standard table:

`P(-0.96<z<1.27)=P(z<1.27)-P(z<-0.96)= 0.898-0.169 = 0.729`