Question

A 4.215 g sample of a compound containing only carbon, hydrogen, and oxygen is burned in an excess of oxygen gas, producing 9.582 g CO2 and 3.922 g H2O. What percent by mass of oxygen is contained in the original sample?

Answer 1

`\% O=27.6\%`

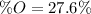
Step-by-step explanation:

Hello,

In this case, for the sample of the given compound, we can compute the moles of each atom (carbon, hydrogen and oxygen) that is present in the sample as shown below:

- Moles of carbon are contained in the 9.582 grams of carbon dioxide:

`n_C=9.582gCO_2*(1molCO_2)/(44gCO_2)*(1molC)/(1molCO_2) =0.218molC`

- Moles of hydrogen are contained in the 3.922 grams of water:

`n_H=3.922gH_2O*(1molH_2O)/(18gH_2O) *(2molH)/(1molH_2O) =0.436molH`

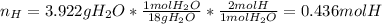
- Mass of oxygen is computed by subtracting both the mass of carbon and hydrogen in carbon dioxide and water respectively from the initial sample:

`m_O=4.215g-0.218molC*(12gC)/(1molC) -0.436molH*(1gH)/(1molH) =1.163gO`

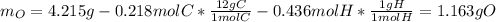
Finally, we compute the percent by mass of oxygen:

`\% O=(1.163g)/(4.215g)*100\% \\\\\% O=27.6\%`

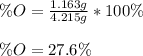
Regards.