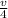Question

To understand how to find the velocities of objects after a collision.

There are two main types of collisions that you will study: perfectly elastic collisions and perfectly inelastic collisions. When two objects collide elastically, both total kinetic energy and total momentum are conserved. These two conservation laws allow the final motion of the two objects to be determined. When two objects collide inelastically, total momentum is conserved, but the total kinetic energy is not conserved. After an inelastic collision the two objects are stuck together, and thus travel with the same final velocity; this fact, together with conservation of momentum, allows the final motion of the two objects to be calculated.
In reality, there is a range of collision types, with elastic and perfectly inelastic at the extreme ends. These extreme cases allow for a more straightforward analysis than the in-between cases. The applet at the end of the problem will give you a chance to explore the "in-between" collisions.
Let two objects of equal mass collide. Object 1 has initial velocity , directed to the right, and object 2 is initially stationary.
Part A
If the collision is perfectly elastic, what are the final velocities and of objects 1 and 2?
Give the velocity v_1 of object one, followed by object v_2 of object two, seperated by a camma. Express each velocity in terms of v.
Part B
Now suppose that the collision is perfectly inelastic. What are the velocities v_1 and V_2 of the two objects after the collision.
Give the velocity v_1 of object one, followed by object v_2 of object two, seperated by a camma. Express each velocity in terms of v.
Part C
Now assume that the mass of object 1 is 2m, while the mass of object 2 remains m . If the collision is elastic, what are the final velocities v_1 and v_2 of objects 1 and 2?
Give the velocity v_1 of object one, followed by object v_2 of object two, seperated by a camma. Express each velocity in terms of v.
Part D
Let the mass of object 1 be and the mass of object 2 be . If the collision is perfectly inelastic, what are the velocities of the two objects after the collision?
Give the velocity v_1 of object one, followed by object v_2 of object two, seperated by a camma. Express each velocity in terms of v.

Answer 1

There are some information missing on Part D: Let the mass of object 1 be m and the mass of object 2 be 3m. If the collision is perfectly inelastic, what are the velocities of the two objects after the collision? Give the velocity v_1 of object one, followed by object v_2 of object two, separated by a comma. Express each velocity in terms of v.

Answer: Part A: v_1 = 0; v_2 = v

Part B: v_1 = v_2 =
`(v)/(2)`

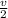
Part C: v_1 =
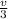`(v)/(3)`; v_2 =
`(4v)/(3)`

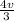
Part D: v_1 = v_2 =
`(v)/(4)`

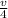
Explanation: In elastic collisions, there no loss of kinetic energy and momentum is conserved. Momentum is determined as p = m.v and kinetic energy as K =
`(1)/(2)`m.
`v^(2)`

Conserved means that the amount of initial momentum is equal to the amount of final momentum:

`m_(1)`.
`v_(1i)` +
`m_(2)`.
`v_(2i)` =
`m_(1).v_(1f) + m_(2).v_(2f)`

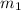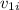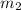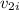No loss of energy means that initial kinietc energy is the same as the final kinetic energy:

`(1)/(2)(m_(1).v_(1i) + m_(2).v_(2i)) = (1)/(2) (m_(1).v_(1f) + m_(2).v_(2f) )`

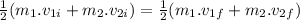
To determine the final velocities of each object, there are 2 variables and two equations, so working those equations, the result is:

`v_(2f) = \frac{2.m_(1) } {m_(1) + m_(2) }.v_(1i) + ((m_(2) - m_(1)))/(m_(1) + m_(2) ) . v_(2i)`

`v_(1f) = (m_(2) - m_(1) )/(m_(1) + m_(2) ) . v_(1i) + (2.m_(2) )/(m_(1) + m_(2) ) .v_(2i)`

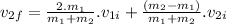
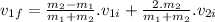
For all the collisions, object 2 is static, i.e.
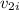`v_(2i)` = 0

Part A: Both objects have the same mass (m),
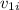`v_(1i)` = v and collision is elastic:

v_1 =
`(m_(2) - m_(1))/(m_(1) + m_(2) ) . v_(1i)`

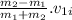
v_1 = 0

v_2 =
`(2.m_(1) )/(m_(1) + m_(2)).v_(1i)`

v_2 =
`(2.m)/(m+m).v`

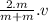
v_2 = v

When the masses are the same and there is an object at rest, the object in movement stops and the object at rest has the same same velocity as the object who hit it.

Part B: Same mass but collision is inelastic: An inelastic collision means that after it happens, the two objects has the same final velocity, then:

`m_(1)`.
`v_(1i)` +
`m_(2)`.
`v_(2i)` =
`m_(1).v_(1f) + m_(2).v_(2f)`

`m_(1).v_(1i) = (m_(1)+m_(2)).v_(f)`

`v_(f) = (m_(1).v_(1i))/(m_(1) + m_(2) )`

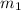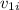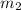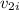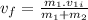
v_1 = v_2 =
`(m.v)/(m+m)`

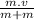
v_1 = v_2 =
`(v)/(2)`

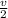
Part C: Object 1 is 2m, object 2 is m and elastic collision:

v_1 =
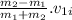
`(m_(2) - m_(1))/(m_(1) + m_(2) ) . v_(1i)`

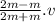
v_1 =
`(2m - m)/(2m + m ) . v`

v_1 =
`(v)/(3)`

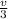
v_2 =
`(2.m_(1) )/(m_(1) + m_(2)).v_(1i)`

v_2 =
`(2.2m)/(2m+m).v`

v_2 =
`(4v)/(3)`

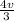
Part D: Object 1 is m, object is 3m and collision is inelastic:

v_1 = v_2 =
`v_(f) = (m_(1).v_(1i))/(m_(1) + m_(2) )`

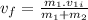
v_1 = v_2 =
`(m)/(m+3m).v`

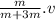
v_1 = v_2 =
`(v)/(4)`