Answer:
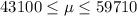
And for this case we want to test the following hypothesis:
Null hypothesis:
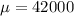
Alternative hypothesis:
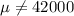
For this case since the lower value of the confidence interval is higher than 42000 we have enough evidence to reject the null hypothesis at the 55 of significance and we can conclude that the true mean is significantly different from 42000
Explanation:
The confidence interval for the mean is given by the following formula:
 (1)
(1)
And for this case the 95% confidence interval is already calculated as:
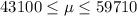
And for this case we want to test the following hypothesis:
Null hypothesis:
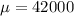
Alternative hypothesis:
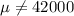
For this case since the lower value of the confidence interval is higher than 42000 we have enough evidence to reject the null hypothesis at the 55 of significance and we can conclude that the true mean is significantly different from 42000