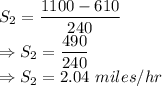Question

PLEASE HELP!!! Two towns are 1100 miles apart. A group of hikers starts from each town and walks down the trail toward each other. They meet after a total hiking time of 240 hours. If one group travels one half mile per hour slower than the other​ group, find the rate of each group.

Answer 1

2.54 miles/hr

2.04 miles/hr

Explanation:

Given: Distance between the two towns = 1100 miles

Difference between speeds =
`(1)/(2)` miles/hr

Total time when they meet = 240 hours

To find:

Let distance traveled by first group =
`x` miles.

Now, the distance traveled by second group = (1100-
`x`) miles/hr

The relation between Speed, Time and Distance is given as:

`\text{Speed =} \frac{\text{Distance}}{\text{Time}}`

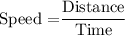
Let speed of first group =
`S_1`

`S_1 = (x)/(240)`

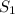
Let speed of second group =
`S_2`

`S_2 = (1100-x)/(240)`

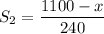
As per question,
`S_1` =
`S_2` +
`(1)/(2)`

`(x)/(240) = (1100-x)/(240) + (1)/(2)\\\Rightarrow (x)/(240) - (1100-x)/(240) = (1)/(2)\\\Rightarrow (x-1100+x)/(240) = (1)/(2)\\\Rightarrow 2x - 1100 = 120\\\Rightarrow 2x = 1220\\\Rightarrow x = 610 ft`

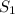Now,

`S_1 = (x)/(240)`

Putting value of
`x`:

`S_1 = (610)/(240)\\\Rightarrow S_1 = 2.54\ miles/hr`

Similarly, putting value of
`x` in
`S_2`:

`S_2 = (1100-610)/(240)\\\Rightarrow S_2=(490)/(240)\\\Rightarrow S_2 = 2.04\ miles/hr`