Question

The amounts of time per workout an athlete uses a stairclimber are normally​ distributed, with a mean of 24 minutes and a standard deviation of 7 minutes. Find the probability that a randomly selected athlete uses a stairclimber for​

(a) less than 19 ​minutes,
(b) between 24 and 33 ​minutes, and​
(c) more than 40 minutes.

Which event is unusual?

Answer 1

To find the probabilities of an athlete using a stairclimber for various times, we calculate the corresponding Z-scores and look up the probabilities in a standard normal distribution table, identifying events beyond 2 standard deviations from the mean as unusual.

Step-by-step explanation:

To find the probability that a randomly selected athlete uses a stairclimber for different intervals of time, we need to use the properties of the normal distribution, which has a mean (μ) of 24 minutes and a standard deviation (σ) of 7 minutes.

Calculating Probabilities

(a) To find the probability of less than 19 minutes, we calculate the Z-score for 19 and look up the corresponding probability in a standard normal distribution table

(b) To find the probability between 24 and 33 minutes, we find the Z-scores for both values and then determine the probability between these Z-scores.

(c) To find the probability of more than 40 minutes, we calculate the Z-score for 40 and subtract the corresponding cumulative probability from 1.

Events more than 2 standard deviations from the mean (in this case, beyond 38 minutes or less than 10 minutes) are considered unusual in a normal distribution.

Answer 2

(a) The probability that a randomly selected athlete uses a stairclimber for​ less than 19 ​minutes is 0.2388.

(b) The probability that a randomly selected athlete uses a stairclimber for​ between 24 and 33 ​minutes is 0.3997.

(c) The probability that a randomly selected athlete uses a stairclimber for​ more than 40 minutes is 0.0113.

Step-by-step explanation:

We are given that the amounts of time per workout an athlete uses a stairclimber are normally​ distributed, with a mean of 24 minutes and a standard deviation of 7 minutes.

Let X = amounts of time per workout an athlete uses a stairclimber

So, X ~ Normal(
`\mu=24,\sigma^(2) =7^(2)`)

The z-score probability distribution for the normal distribution is given by;

Z =
`(X-\mu)/(\sigma)` ~ N(0,1)

where,
`\mu` = mean time = 24 minutes

`\sigma` = standard deviation = 7 minutes

(a) The probability that a randomly selected athlete uses a stairclimber for​ less than 19 ​minutes is given by P(X < 19 minutes)

P(X < 19 min) = P(
`(X-\mu)/(\sigma)` <
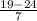`(19-24)/(7)` ) = P(Z < -0.71) = 1 - P(Z
`\leq` 0.71)

= 1 - 0.7612 = 0.2388

The above probability is calculated by looking at the value of x = 0.71 in the z table which has an area of 0.7612.

(b) The probability that a randomly selected athlete uses a stairclimber for​ between 24 and 33 ​minutes is given by = P(24 min < X < 33 min)

P(24 min < X < 33 min) = P(X < 33 min) - P(X
`\leq` 24 min)

P(X < 33 min) = P(
`(X-\mu)/(\sigma)` <
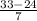`(33-24)/(7)` ) = P(Z < 1.28) = 0.8997

P(X
`\leq` 24 min) = P(
`(X-\mu)/(\sigma)`
`\leq`
`(24-24)/(7)` ) = P(Z
`\leq` 0) = 0.50

The above probability is calculated by looking at the value of x = 1.28 and x = 0 in the z table which has an area of 0.8997 and 0.50 respectively.

Therefore, P(24 min < X < 33 min) = 0.8997 - 0.50 = 0.3997

(c) The probability that a randomly selected athlete uses a stairclimber for​ more than 40 minutes is given by P(X > 40 minutes)

P(X > 40 min) = P(
`(X-\mu)/(\sigma)` >
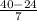`(40-24)/(7)` ) = P(Z > 2.28) = 1 - P(Z
`\leq` 2.28)

= 1 - 0.9887 = 0.0113

The above probability is calculated by looking at the value of x = 2.28 in the z table which has an area of 0.9887.

The event of probability that a randomly selected athlete uses a stairclimber for​ more than 40 minutes is unusual because this probability is less than 5% and any even whose probability is less than 5% is said to be unusual.