Question

What is the Volume of the square pyramid? (Round to the nearest tenth as needed)

Answer 1

5229 mm³

Explanation:

Volume of square pyramid

`\sf \boxed{Volume \ of \ the \ square \ pyramid = (1)/(3)*b^2*H}`

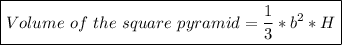
b = base length = 25 mm

H = height

We have to find 'H' using Pythagorean theorem,

slant height (hypotenuse) = 28 mm

leg₁ = base length ÷ 2 = 25÷2 = 12.5

leg₂ = H

H² + (12.5)² = 28²

H² = 784 - 156.25

= 627.75

`\sf H = √(627.75)`

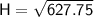
H = 25.01 mm

`\sf \text{Volume of square pyramid =$(1)/(3)*25*25*25.1$ }`

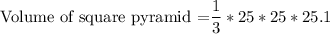
= 5229 mm³

`\sf \boxed{\text{Volume of square pyramid = (1)/(3)*25*25*28}}`