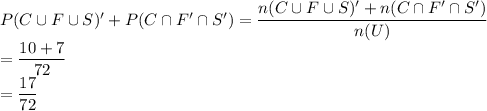Answer:
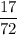
Explanation:
From the given information:
Total number of students,
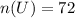
The choices were: Chess(C), Swimming(S), and Football(F).
Everyone liked at least one sport except 7 kids,

Chess is not an active sport; and
10 children liked Chess only,

The probability that a randomly-chosen child from this group does not like active kinds of sport is the Probability that a student plays chess only or like no kind of sport at all.