Answer:
8.15% probability your hand contains exactly 3 hearts
Explanation:
A probability is the number of desired outcomes divided by the number of total outcomes.
The order in which the cards are chosen is not important, so we use the combinations formula to solve this question.
Combinations formula:
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

What is the probability your hand contains exactly 3 hearts (out of 5 cards)?
We have 52 cards in total.
13 are clubs, 13 are spades, 13 are hearts, 13 are diamonds.
Desired outcomes:
3 hearts, from a set of 13.
Other 2 cards, from a set of 52-13 = 39.
So
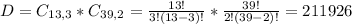
Total outcomes:
5 cards, from a set of 52. So
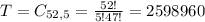
Probabilities:
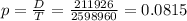
8.15% probability your hand contains exactly 3 hearts