Question

A 60-kg skier is stationary at the top of a hill. She then pushes off and heads down the hill with an initial speed of 4.0 m/s. Air resistance and the friction between the skis and the snow are both negligible. How fast will she be moving after she is at the bottom of the hill, which is 10 m in elevation lower than the hilltop

Answer 1

The Skier's velocity at the bottom of the hill will be 18m/s

Step-by-step explanation:

This is simply the case of energy conversion between potential and kinetic energy. Her potential energy at the top of the hill gets converted to the kinetic energy she experiences at the bottom.

That is

`mgh = 0.5 mv^(2)`

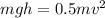
solving for velocity, we will have

`v= √(2gh)`

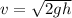
hence her velocity will be

`v=√(2 * 9.81 * 10)=14.00m/s`

This is the velocity she gains from the slope.

Recall that she already has an initial velocity of 4m/s. It is important to note that since velocities are vector quantities, they can easily be added algebraically. Hence, her velocity at the bottom of the hill is 4 + 14 = 18m/s

The Skier's velocity at the bottom of the hill will be 18m/s

Answer 2

The velocity is
`v = 8.85 m/s`

Step-by-step explanation:

From the question we are told that

The mass of the skier is
`m_s = 60 \ kg`

The initial speed is
`u = 4.0 \ m/s`

The height is
`h = 10 \ m`

According to the law of energy conservation

`PE_t + KE_t = KE_b + PE_b`

Where
`PE_t` is the potential energy at the top which is mathematically evaluated as

`PE_t = mg h`

substituting values

`PE_t = 60 * 4*9.8`

`PE_t = 2352 \ J`

And
`KE_t` is the kinetic energy at the top which equal to zero due to the fact that velocity is zero at the top of the hill

And
`KE_b` is the kinetic energy at the bottom of the hill which is mathematically represented as

`KE_b = 0.5 * m * v^2`

substituting values

`KE_b = 0.5 * 60 * v^2`

=>
`KE_b = 30 v^2`

Where v is the velocity at the bottom

And
`PE_b` is the potential energy at the bottom which equal to zero due to the fact that height is zero at the bottom of the hill

So

`30 v^2 = 2352`

=>
`v^2 = (2352)/(30)`

=>
`v = \sqrt{ (2352)/(30)}`

`v = 8.85 m/s`